A "new" analytic solution to a classic solar wind problem?

This web page gives an overview of results that have been published in the November 2004 issue of the American Journal of Physics. (Here is a link to the preprint at the arXiv e-print server;) here is a local link to a PDF reprint.) This page was written with a general audience in mind, but please forgive me (and let me know) if I slip into too much jargon!

What I've derived is an exact, closed-form, analytic solution to Parker's isothermal solar wind equation. To those who've never heard of this equation, let me just say that in 1958, Eugene Parker of the University of Chicago published a ground-breaking paper wherein he predicted that the Sun continuously ejects gas (i.e., the "solar wind"), and this gas starts out at low speeds close to the Sun and accelerates to supersonic speeds - exceeding 1 million miles per hour - far from the Sun. His paper presented the equations that describe this acceleration along with preliminary solutions of the equations. There was only one problem: even for the simplest possible conditions that one could assume about the outer solar atmosphere (i.e., that its temperature is constant, or "isothermal") it was not possible to use algebra to solve the governing equations for the wind speed as a function of distance from the Sun. One needs to use numerical methods, most often implemented using computers, to solve these equations.

To those familiar with the classic Parker problem, it might come as a surprise to find out that one can solve the transcendental equation in closed form. This is done by using a relatively new special function called the Lambert W function that was designed specifically to allow explicit analytic solutions to a wide class of equations that were only previously considered to be solvable implicitly.

My colleagues might also (rightly) ask: "Why bother?" since the state-of-the-art in solar wind physics has moved far beyond the relatively simple isothermal Parker problem. Well, theorists can argue that the sheer elegance of having an explicit analytic solution is worth the effort. However, there could be practical benefits as well. Analytic expressions are often used as initial guesses for more complicated iterative, time-dependent, or multi-dimensional calculations. Closed-form solutions also make it easier to study linearized perturbations to a "known" background state. The rapid evaluation of a large number of cases is made easier by having analytic formulae, especially since many symbolic computation packages (like Mathematica, Maple, etc.) already contain optimized routines for the Lambert W function. Finally, the ability to write down simple expressions for solar wind plasma properties may make the extrapolation to other stars more tractable and physically understandable.

My recently published journal paper also discusses another related problem that can be solved analytically by using the Lambert W function: how the solar mass loss rate (i.e., how many kg of solar gas is expelled per second) is determined. I won't go into this in any more detail on this web page and just refer the curious reader to the paper (PDF).

The Lambert W function

Let's start with a simple example. Can you solve this equation for the variable x ?

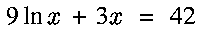
No? If you make a graph of the left-hand-side versus x, you find that it crosses the "right-hand" value of 42 when x equals about 7.827189. You can also plug in this value and verify it with a calculator. Looking at the problem this way gives you a so-called "implicit" solution, but it's just not as satisfying as being able to use algebra to move things around so that x is by itself on one side of the equation, and something else that you can evaluate simply (the "explicit" solution) is on the other side of the equation.

The Lambert W function (named after 18th century mathematician Johann Lambert who laid the groundwork for the modern work that defined the function) was designed specifically to let us solve an equation like the above for x. The function W(x) is formally defined as the multi-valued inverse of the function: x times exp(x). In other words, W(x) is the solution to the following equation:

In practice, one can just make a big list of values of W, then compute x for each value, and then use it as a "reverse look-up table" to locate the value of W that corresponds to any desired x. Care must be taken when x is negative, though, because then there are either two solutions for W (called the two principal branches) or none. Here is a plot of the two principal branches of W(x), labeled with subscripts 0 and -1:

Lots more can be found about the Lambert W function on Robert Corless' home page or at the great Weisstein/Wolfram site MathWorld. There is also an introductory article by Brian Hayes titled "Why W?" in the March-April 2005 issue of American Scientist.

To make a long story short, I am indebted to Kieth Briggs who summarized a very useful way of finding solutions to many equations using the Lambert W function. Briggs noted that the following equation:

has the general solution in terms of W:

The number of solutions depends on the number of branches of W(x) that exist for the specified argument of the function. Thus, the analytic solution to the above example is:

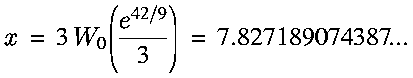
and we knew to choose the '0' branch because the argument of the function is positive (see the above figure).

The Isothermal Solar Wind Problem

If you've read this far, I won't bore you with too much extra derivation; you can find more details in the paper. The isothermal Parker solar wind equation is written implicitly as:

where v is the outflow velocity of the wind, which is the quantity we wish to solve for, r is the distance (measured here from the center of the Sun), a is the speed of sound in the outer solar atmosphere, which is proportional to the temperature of the gas, and which we assume to be constant. Also, rc is the so-called "Parker critical-point distance" where the wind accelerates past the sound speed:

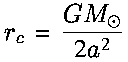
where G is Newton's gravitational constant, and M is the mass of the Sun (the funny subscript is the symbol for the Sun).

Traditionally, it has been considered not possible to solve the above big equation for v. But it is possible with the Lambert W function. For the solution branch that applies to the solar wind, we get:

where the argument of the Lambert W function is:

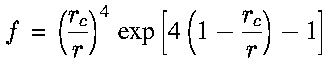
Lots more can be said about the mathematical properties of this solution. Note that one has to switch solution branches at the critical point. The opposite choice of the W(x) branches gives an explicit analytic solution for the so-called Bondi spherical accretion problem, which was first worked out in 1952 (see another ground-breaking paper), but similarly no exact, explicit solution was known before now.

To summarize, here is a plot of the various solutions, including others that do not correspond to physically realistic flows, but can also be solved for using the Lambert W function. The (red) Parker solar wind solution is labeled as "transonic wind" because it goes from subsonic to supersonic; the Parker critical point is labeled in yellow.

The Lambert W function is an interesting new "hammer" in our toolbox. Here is a brief list of additional problems in solar physics and astronomy that can be pounded on with this hammer:

See my paper for references to other papers that use the Lambert W function to solve problems in electrostatics, statistical mechanics, general relativity, radiative transfer, quantum chromodynamics, combinatorial number theory, fuel consumption, and population growth. The Wikipedia article on the Lambert W function has more information, too. (Thanks to Tony Scott!)

Finally, if you end up using the Lambert W function in some new application, I would suggest letting Bob Corless, the main author of one of the first and most-cited papers on the Lambert W function, know about it.

GO BACK TO: Steven Cranmer's home page